Let’s Have S’more Chemistry: Marshmallows, Chocolate, Grams, and Moles
Contributed by OLU: Tanya Grasz.
Tanya Grasz teaches Honors Chemistry. Students who enroll for this class have demonstrated solid mastery of biology and algebra as well as a capacity for problem solving and critical thinking. The subject matter is demanding. Even well-prepared students often struggle with the advanced content as concepts build and converge over time with increasingly complex applications.
Ms. Grasz approaches this challenge with the same spirit of inquiry she expects from her students as they engage in ongoing investigation of scientific phenomenon. She is energized when students grasp an important lesson or idea but also perplexed when they struggle to master key concepts after careful teaching and assistance. One example is her ongoing efforts to help students use mole ratios when solving stoichiometry problems.
Brief Intro to Stoichiometry
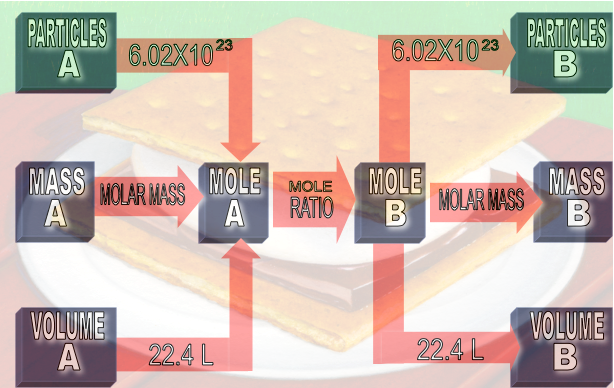
Equations are a chemist’s recipe. Equations tell chemists what amounts of reactants to mix and what amounts of products to expect. When you know the quantity of one substance in a reaction, you can calculate the quantity of any other substance consumed or created in the reaction. For example, if we have the reaction N2 + 3H2 → 2NH3, we know that nitrogen and hydrogen molecules will react in a 1:3 proportion. The calculation of quantities in chemical reactions is called stoichiometry and mole ratios are the bridge for converting between various units of chemical quantities.
Teaching and Learning Challenge
Ms. Grasz found that students typically struggle with several key aspects of solving stoichiometry problems as well as general principles of dimensional analysis:
Interpreting and setting up problems
Understanding where the problem resides on the “mole map” (realizing what they are given and understanding where they need to end up)
Understanding and using mole ratios as the critical bridge for converting units of one substance to units of another.
And, most importantly...moving from a formulaic pattern (calculations of units) to a conceptual understanding of mole ratios.
Bridging the Gap
Ms. Grasz teamed up with another science colleague, Jill Ronstadt, to investigate instructional solutions. They planned and implemented a research lesson with the following components.
First, they used a cooking analogy to help students grasp the proportional conversion process with something familiar and accessible from everyday life. “A recipe is like a balanced chemical equation,” Ms. Grasz explained. “Ingredients are the reactants. Cookies or cake or burritos are the products.”
She then asked students to think through an example recipe for S’mores. The goal was for the students to understand that there is no way to know how many S’mores could be made given a certain number of ingredients if there is no recipe to follow or refer to.
Moving from food to chemistry, Ms. Grasz helped students review what the coefficients in the chemical equations represent using a relatively simple equation for ammonia (NH3) production. They discussed why the coefficients do not represent grams, but instead represent moles and molecules or sometimes liters (for problems using gases at standard temperature and pressure). This is why using mole ratios are so important--they bridge the gap between various units in the conversion process.
Next, Ms. Grasz asked students to work on a stoichiometry problem. The problem asked students to move from grams to moles. “How many grams of ammonia are produced from 0.60 moles N2? “ She gave students time to work individually. While they were working, she took inventory of students that solved the problem successfully and those that made a wrong turn somewhere in the process. She found that 8 of 27 students had solved the problem correctly. She then divided the class into eight groups and asked students who were successful to teach and discuss with others how they solved the problem. Ms. Grasz wrapped up the lesson by reminding students that the equation is their “recipe” and the numbers for the mole ratio are coming from “the coefficients of a balanced chemical equation.” She then assigned additional problems for homework, including several questions about a recipe for chocolate chip cookies, and two problems involving chemical equations.
Video Clip #2 - Practice with Stoichiometry
Day 2 Results
The results from homework showed that nearly all students were able to solve problems about chocolate chip cookies, but most still had questions about problems using chemical equations. “They knew the mantra about using their equation to find the mole ratios,” Ms. Grasz explained, “but had not connected all the dots yet.”
Ms. Grasz spent the next class period persisting to help all students “connect the dots” and learn to use the balanced chemical equation as their “recipe” for solving stoichiometry problems. She guided the class through the homework examples. She paused at each phase of the problems to elicit student thinking and asked them to direct the solution process. She then gave students a new problem to solve independently and circulated around the room to document student progress. While circulating, she strategically placed a dot on individual papers to keep track of where students were struggling or asking questions. She then collected the papers to better diagnose the primary areas of difficulty. During this second round of work, Ms. Grasz’s records showed that of 27 students in the class, all but 8 solved the problem successfully and 5 of these 8 failed to correctly use the mole ratio. This was a substantial improvement from Day 1 where only eight students solved the Ammonia problem correctly. Ms. Grasz continued with several more problems at the end of the period until the entire class could use the “recipes” effectively.
The excerpts below from student interviews describe this journey of discovery and deeper understanding from two students’ point of view. Both students were members of the same small group featured in the second video clip above. In the video, Cove was the “teacher” for the group. Brandon was the student on the far right, opposite side of the table.
Interview with honors chemistry student, Brandon Washiashi
I: When you were first asked to work on that ammonia problem, what process did you use to solve the problem. Where did you start?
B: We were coming off the previous test which covered moles and if I remember correctly, I think I tried taking ammonia and turning into moles…
I: How did that work for you?
B: Well, I got the wrong answer.
I: What were you were struggling with?
B: The numbers...I didn’t know the ratio and it’s kind of hard to calculate when you don’t know how much to calculate.
I: What did you understand about the s’mores analogy at that point?
B: I didn’t think to look there...It wasn’t on my radar until after the revelation of the ammonia problem.
I: What did you learn from the conversation with Cove and the group work?
B: That I need the correct amount of coefficients to make the correct number of ammonia.
I: After that, you had some homework problems. How were those?
B: The first one I was still getting into...I still had a couple of questions...after that I’ve been fine.
I: Was there anything that Cove said, that Mrs. Grasz said...where did it start to “click” for you?
B: I used the map...that was a big help...making sure it’s a balanced equation, of course, plug it in, and follow the bridge. For me, it was pretty straight-forward after that.
I: Where do you think your biggest gap was prior to that?
B: All goes back to the ratio...I can can’t cross unless I know the ratio.
I: How is this class affecting the way you think about science?
B: Well, I’m definitely thinking about it more mathematically now...Knowing all of the previous stuff is helping, because if I didn’t know how to look at a periodic table, I wouldn't be able to find the atomic mass of Oxygen. If I didn’t know Oxygen was a diatomic molecule I would just look at it as...one atom instead of two. If I didn’t know about moles, I couldn’t convert into moles and cross the bridge and figure out the problem.
Interview with honors chemistry student, Cove Carlson
I: What were you thinking as you listened to Ms. Grasz explain the s’mores analogy?
C: We just finished a unit on conversion factors...I was comparing it to [moles]...I was piecing together as well, the balanced equation--the coefficients played a part...It was easy to picture the ratio. I make s’mores...so I can picture how each one corresponds to the other.
I: When you were first asked to work on that ammonia problem, tell me about your thinking.
C: I’m not a very good math person, so I know I was a little nervous. I wasn’t expecting to get it right, but I did have a sensation that “this makes sense”….I was looking at what I was given and thinking back to what she taught us with s’mores...There’s 0.5 chocolate bars to two graham crackers...there’s two ammonia to whatever it was in the equation…
I: Was there anything in particular that Ms. Grasz said that helped you?
C: It really stuck with me that the coefficients are an important part of the balanced chemical equation.
I: During the group work segment, how did you feel about teaching the group?
C: ...I felt a little proud...I got this. It makes sense to me.
I: Can you remember more about what was making sense to you?
C: ...Before it had always been one mole. This equals one mole; one mole equals this. The biggest thing I understood was that now it wasn’t one mole. It was two, three, four, however many--the coefficients of the chemical equation.
I: How is this class affecting the way you think about science?
C: Before going into this class, I was thinking, “I’m not a science person...” After this, I was grounded. This is a solid base for me. I know how to do this. I can keep building off this...You got to be persistent...You might spend three or four hours on an assignment, but if that helps you get it, everything else becomes so easy, because it all builds off each other.
Reflections
As illustrated in the student comments above, successful classroom teaching is rarely a silver bullet or single magical strategy. More often, it’s the hard work of carefully stringing together a sequence of subtle, incremental innovations that help students advance toward deeper understanding. Ms. Grasz’s stoichiometry work is a great example. She helped students access prior knowledge and make connections to the work done in previous chapters. She developed a compelling analogy with the s’mores recipe. She strategically grouped students so they could learn from each other’s problem-solving efforts. She gave them multiple opportunities for practice and feedback. She diagnosed where they were struggling and scaffolded their learning through guided questions and targeted assistance. She helped students remember to start with their recipe--the balanced chemical equation--and she helped them learn to use the “mole ratio” as the bridge between reactants and products.
Ms. Grasz’s persistence with teaching fueled a parallel persistence with learning, enabling students to grapple with difficult problems and master core concepts of stoichiometry. Ms. Grasz and Ms. Ronstadt are now mapping out their next project to study and improve teaching and learning. Stay tuned for s’more examples and findings from teaching and learning at OLu.